Theorem
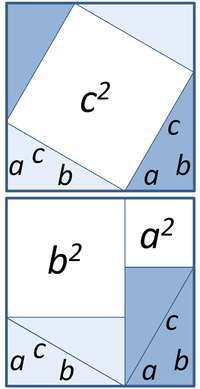
A theorem is a proven idea in mathematics. Theorems are proved using logic and other theorems that have already been proved. A minor theorem that one must prove to prove a major theorem is called a lemma.[2] Theorems are made of two parts: hypotheses and conclusions.
Theorems use deduction, in contrast to theories which are empirical.[3]
Some theorems are trivial, since they directly follow from the propositions. Other theorems are called "deep", because their proof is long and difficult. Sometimes, such proofs involve other areas of mathematics or show connections between different areas.[4] A theorem might be simple to state and yet be deep. An excellent example is Fermat's Last Theorem, and there are many other examples of simple yet deep theorems in number theory and combinatorics (among other areas).
There are other theorems for which a proof is known, but cannot be easily written down. Among the best examples are the four color theorem and the Kepler conjecture. Both of these theorems are only known to be true by reducing them to a computational search which is then verified by a computer program. At first, many mathematicians did not accept this form of proof, but it has become more widely accepted over the years. The mathematician Doron Zeilberger has even gone so far as to claim that these are possibly the only nontrivial results that mathematicians have ever proved.[5] Many mathematical theorems can be reduced to simpler computations, including polynomial identities, trigonometric identities and hypergeometric identities.[6]
Related pages
[change | change source]References
[change | change source]- ↑ For full text of 2nd edition of 1940, see Elisha Scott Loomis. "The Pythagorean proposition: its demonstrations analyzed and classified, and bibliography of sources for data of the four kinds of proofs" (PDF). Education Resources Information Center. Institute of Education Sciences (IES) of the U.S. Department of Education. Retrieved 2010-05-04. Originally published in 1940 and reprinted in 1968 by National Council of Teachers of Mathematics.
- ↑ "The Definitive Glossary of Higher Mathematical Jargon". Math Vault. 2019-08-01. Retrieved 2020-10-10.
- ↑ However, both theorems and theories are investigations. See Heath 1897 Introduction, The terminology of Archimedes, p. clxxxii:"theorem (θεὼρνμα) from θεωρεἳν to investigate"
- ↑ Weisstein, Eric W. "Deep Theorem". mathworld.wolfram.com. Retrieved 2020-10-10.
- ↑ "Doron Zeilberger's 51st Opinion". sites.math.rutgers.edu. Retrieved 2020-10-10.
- ↑ Petkovsek et al. 1996.
Books
[change | change source]- Heath, Sir Thomas Little (1897), The works of Archimedes, Dover, retrieved 2009-11-15
- Hoffman, P. (1998). The Man Who Loved Only Numbers: The Story of Paul Erdős and the Search for Mathematical Truth. Hyperion, New York.
- Petkovsek, Marko (2019). A = B. AK Peters. ISBN 978-0-367-44871-4. OCLC 1129744105.
